The special triangle
Thousands of years ago, in ancient Egypt, there were men known as
“rope-stretchers.” It was their job to mark out the boundaries of fields
and the foundations for houses, temples, and palaces.
The ancient Egyptians preferred square and rectangular fields and
buildings, with perfectly

square corners. But they didn’t have all the special measuring tools we
have today. So how do you suppose the rope-stretchers were able to make
perfectly square corners? Well, they did it with a special triangle.
To make a square corner, the rope-stretchers used a long piece of rope
tied into a loop. Tied in the loop were twelve equally spaced knots,
just like the marks on a ruler.
First the rope-stretchers pounded a stake into the ground where they
wanted a corner. They placed one of the knots at this stake. Then they
counted out three knots and pulled the rope tight. Another stake was
pounded into the ground at the third knot.
Going back to the corner stake, they picked up the other part of the
rope and counted out four knots. Holding the fourth knot, they pulled
the rope so that all the sides were tight. Then they pounded a stake
into place at the fourth knot. And, presto, they had a special kind of
triangle with the square corner they needed for a field or a building.
What the rope-stretchers had done was make a right triangle—a triangle
that has one right angle forming a square corner. The trick, of course,
was in knowing which knots to put the stakes at. There were twelve knots
in the loop of rope. So there were also twelve equal spaces.
On one side of the corner, the stretched-out rope had three spaces. On
the other side, it had four spaces. And on the side opposite the corner
there were five spaces. So the sides of the triangle were three, four,
and five spaces long. And anytime you have a triangle with sides having
this three, four, five relationship, it will always be a right
triangle.

Try it yourself and see. Cut a length of string and make thirteen
equally spaced marks on it. Put the two end marks on top of one another
and push a tack through both marks so that the string forms a loop. You
.will now have a loop of string with 12 equal spaces. Push the tack into
a piece of wood or heavy cardboard. This will be your corner.
Count out three marks from the corner. Push a tack through this mark.
Pull the string tight and fasten it to the board. Go back to the corner
tack and count out four marks. Push a tack through this mark. Pull the
string until all three sides are tight. Fasten the tack at the fourth
mark to the board. You now have a special triangle just like the one
made by the Egyptian rope-stretchers!
The Egyptian rope-stretchers weren’t the only ones who knew how to make
a right triangle. People in other parts of the world also knew the
secret. And, in time, some people began to wonder just why this way of
making a right triangle always worked.
One of the people who wondered about this was an ancient Greek named
Pythagoras (pih [thag]{.smallcaps} uh ruhs). Pythagoras made dozens and
dozens of right triangles of different sizes. And when he squared the
sides of the triangles, he discovered something. What he learned can be
shown in picture form, like this:
To square a number, multiply the number by itself. The triangle in the
picture has sides 3, 4, and 5 units long. When you multiply 5 x 5, you
get 25. Then you multiply 4×4} getting 16, and 3×3, getting 9. Count the
little- squares next to each of the triangle’s sides and you’ll see that
there are 25, 16, and 9 of them.
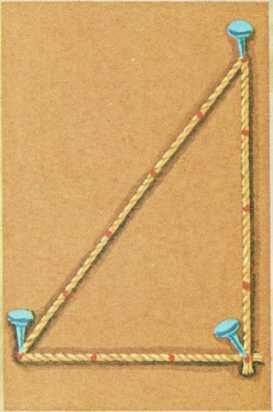

Now if you add the numbers you got by squaring the two smallest
sides—9 and 16— you get 25. So, the number of little squares next to
the two short sides of the triangle add up to the same amount as the
number of squares next to the longest side!
Pythagoras made up a rule about this. The rule says that for a right
triangle the square of the longest side equals the sum of the squares of
the other two sides. The longest side has a special name. It is called
the hypotenuse (hy [paht]{.smallcaps} uh noos), which means
“stretching under.”
This is something that is true of all right triangles. It is why
Egyptian rope-stretchers always got a right triangle with their knotted
rope. As long as they staked out triangles with sides having a three,
four, five relationship, they could only get a right triangle.
What Pythagoras learned may not seem very important, but it is. His rule
helps engineers, mathematicians, astronomers, and others to solve many
different kinds of problems.
Do you belong to a club that has a secret password or a secret symbol?
Some twenty-five hundred years ago, in ancient Greece, there was a kind
of club called The Brotherhood.
This “club” was really a school run by the Greek mathematician
Pythagoras. All of the young men who went to the school studied
mathematics and religion. They took an oath never to reveal the
mathematical secrets they learned. The penalty for giving away a secret
was death!
All the members of The Brotherhood wore a symbol that looked like this:
This shape is called a pentagon [(pehn]{.smallcaps} tuh gahn), which
means “five angles.” Inside the pentagon is another shape, a
five-pointed star called a pentagram [(pehn]{.smallcaps} tuh gram). If
you look closely, you’ll see that in the middle of the star there is a
small pentagon.
The thing that was secret about this symbol was that nobody else knew
how to make it. (If you think it is easy, try it.) Only the members of
The Brotherhood knew how to do it.
They had learned how to make solid shapes, such as cubes and pyramids,
by putting

