The Roads of Math
Take a line, a straight line, And divide it into three.
Make the three lines form three angles, And a triangle have we.


Now take this little triangle And twirl it about in space.
Twirl the triangle ’round and ’round; A cone is what we face.
Now look at the bottom of our cone.
We see a circle true.
Now let’s examine the circle And what it can do for you.

Let’s draw a line through the center Of our circle round.
We’ve discovered something new: The diameter we’ve found.
Let’s look at the line ’round the circle.
The circumference says, “Hi!”
Divide the circumference by the diameter, And we’ve found the number
Pi.^1^

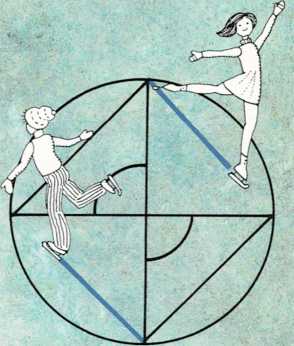
Now take our semicircle
And take a point on the rim. Using the diameter, form a triangle.
(It doesn’t take much vim.)
Look carefully at this triangle.
If you do, something different you’ll see, For the largest of its angles
Has exactly ninety degrees.

Now take two of these new right angles.
Put their vertices ^2^ in the circle’s center, there. With the diameter
under one side of each angle,
We’re ready to form a square.
Mark the points on the circumference Where the right angles do fall.
Construct triangles in both semicircles, And that is all.
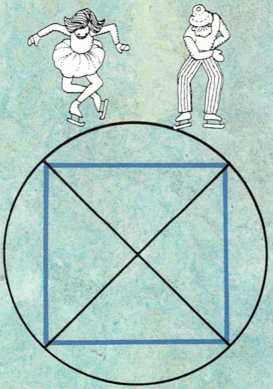
From a line, to a triangle, to a cone, To a circle, to Pi, to a square.
We have traveled the roads of math, Which will take you anywhere!
Jeffrey DieUe
Pi is a Greek letter that looks like this ir. In mathematics, Pi is
a symbol for a number close to 3.14. To find the circumference of a
circle, multiply the diameter by 3.14. And, as the poem says, if you
divide the circumference by the diameter, you get the number 3.14.Vertices [(vur]{.smallcaps} tuh seez) is the plural of vertex. The
vertex is the point at which the sides of an angle come together.
