
The marvelous counting machine
Imagine that it is five thousand years ago and that you are a rich
merchant in the ancient city of Babylon. You have a curly black beard
and a purple robe with yellow fringe at the bottom. You’re sitting in a
sandy yard, in the shade of a clump of palm trees.
You have a problem you need to solve. In your storehouse there are
forty-five baskets of grain. You have just bought forty-three baskets of
grain from a farmer. You want to know how many baskets of grain you now
have.
With your finger, you draw two grooves in the sand. Next to the grooves
you place a small bag full of round, white pebbles. These grooves and
pebbles are actually a marvelous counting- machine you can use to solve
your problem I
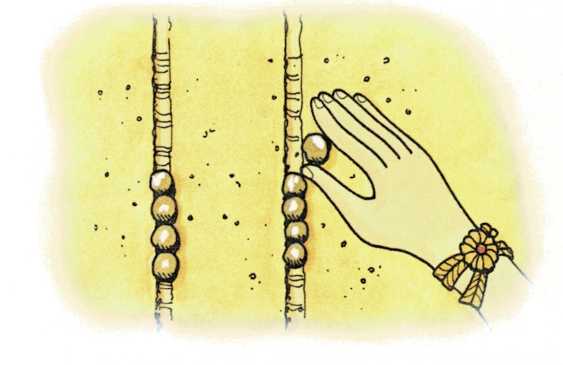
In the right-hand groove you place five of the pebbles. Each of these
pebbles has a value of 1, giving you a total of 5. In the left-hand
groove, you place four pebbles. Each of the pebbles in this groove has a
value of 10, giving you a total of 40. So the pebbles in the two grooves
show that you have four tens (40) plus five ones (5), or a total of
45—the number of baskets of grain in your warehouse.
Now you want to add the forty-three baskets of grain you just bought.
Taking another handful of pebbles from the bag, you place three pebbles
in the ones groove and four pebbles in the tens groove. So you have four
tens (40) plus three ones (3), or a total of 43—the number of baskets
of grain you just bought.
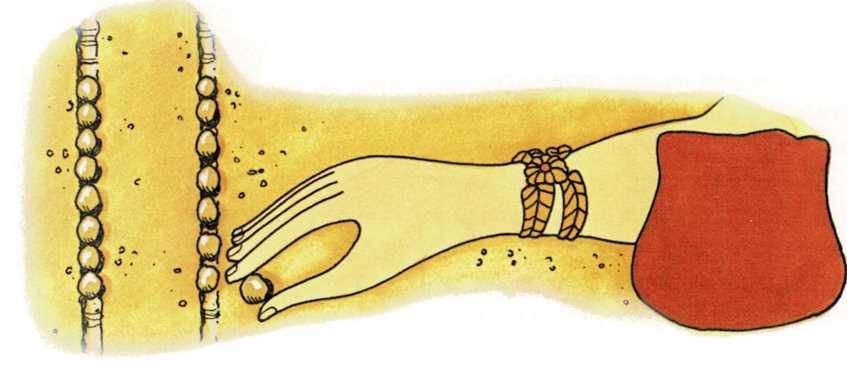
All you have to do now is count the number of pebbles in each groove. In
the left-hand, or tens groove, you have eight pebbles, each with a value
of 10. Eight tens is 80. In the right-hand, or ones groove, you also
have eight pebbles. But each of these pebbles has a value of 1. Eight
ones is 8. So you have 80 + 8, or a total of 88. This is how many
baskets of grain you have.
If you had needed to, you could have drawn more grooves to work out
bigger numbers. If you had drawn a groove to the left of the tens
groove, each pebble you put in it would have a value of 100. And if you
made another groove to the left of the hundreds groove, each pebble in
that groove would have a value of 1,000.
If you think about it, you’ll see that we write our numbers today in
just the same way they were set up on that ancient counting machine. In
a number such as 5,555, the first numeral on the right stands for five
ones (5),
the next numeral to the left stands for five tens (50), the next for
five hundreds (500), and the last numeral on the left for five thousand
(5,000). In a system of this kind, the value of any numeral depends on
where it is placed.
The kind of counting machine an ancient Babylonian merchant used is
called an abacus (AB uh kuhs). Abacus means “slab” or “table.” But
this name comes from an ancient word that means “dust” or “sand.” The
first abacus was probably a tray covered with dust or sand. Counting
marks could be made with a finger and erased with a sweep of the hand.
After a time, people started making grooves in the sand and using
pebbles for counters. Finally, they strung pebbles or beads on wires.
They then had a counting machine that was easy to use and could be
carried around.
The abacus is a tool that was invented by people in almost every part of
the world. The Babylonians and Egyptians used the abacus five thousand
years ago. So did the Chinese. And when the Spaniards came to America,
they found the Maya Indians using the abacus.
When you buy something in a store, you go up to the counter to pay for
it. Have you ever wondered why this table is called a counter? It’s
because, long ago, that’s where merchants used their counting board, or
abacus. Today, that’s where the cash register is. But many shopkeepers
in Asia still use an abacus.
An abacus can be used to work all kinds of arithmetic problems. If you
know how to use it, you can solve problems very quickly. In fact, there
have been contests in which a person using an abacus could do some
problems faster than a person using an electronic calculator!
People used to working with an abacus can do arithmetic as quickly as
someone using a modern counting machine.


